
|
|
Escher wrote back to Coxeter that this figure
“gave me quite a shock,”
since it showed him how to design a
pattern in which the motifs become ever smaller toward a
limiting circle [Co2].
Escher was able to reconstruct the circular arcs in Coxeter's
figure and then use them to create his first circle limit pattern,
Circle Limit I which he included with his letter to Coxeter.
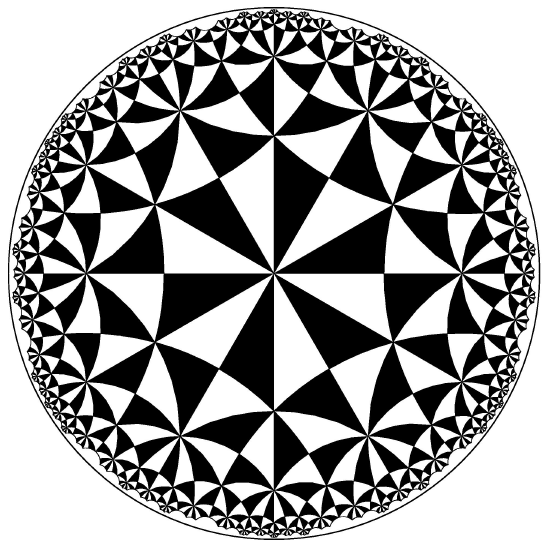
Figure 2 below shows a rough computer rendition of that pattern,
with interior detail for a few of the fish.
It is easy to see the connection between Figures 1 and 2.
Escher also mentioned in his letter
that he had long known of patterns with one internal
limit point, and was familiar with patterns with a limiting line.
Escher's prints Development II (1939) and Smaller and Smaller
(1956) (Catalog numbers 310 and 413 in [Bo1]) and his notebook drawing
number 65 (1944) [Sc1] have single limit points; the last two are
invariant under a similarity.
His prints
Regular Division of the Plane VI (1957)
and
Square Limit (1964)
have line limits (Catalog numbers 421 and 443 [Bo1]).
The points of the Poincaré disk model of hyperbolic geometry
are the interior points of a bounding circle in the Euclidean plane.
In this model,
hyperbolic lines are represented by circular arcs that are
perpendicular to the bounding circle, including diameters.
Figures 1 and 2 show examples of these perpendicular circular arcs.
Equal hyperbolic distances are represented by ever smaller Euclidean
distances as one approaches the bounding circle. For example, all
the triangles in Figure 1 are the same hyperbolic size, as are all the
black fish (or white fish) of Figure 2.
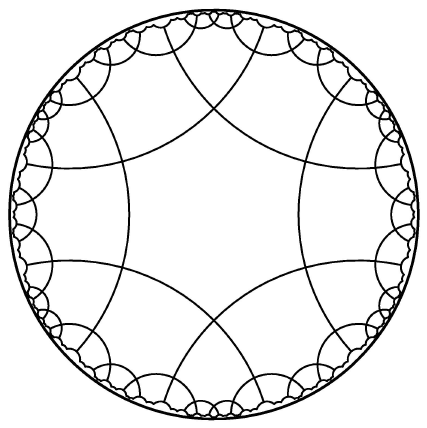
The patterns of Figures 1 and 2
are closely related to the regular hyperbolic tessellation
{6,4} shown in Figure 3 below. In general, {p,q} denotes
the regular tessellation by regular p-sided polygons with q
of them meeting at each vertex.
David Joyce has a web site
Hyperbolic Tessellations
that includes regular, quasi-regular, and star
hyperbolic tessellations [Jo1].
His site has a
Java applet
that allows users to create
their own hyperbolic tessellations.
Martin Deraux also has a web site
Hyperbolic tessellations
with a Java applet that allows users to create different
triangle tessellations such as that of Figure 1 [De1].
Don Hatch has a large array of regular hyperbolic
tessellations at his web site
Hyperbolic Planar Tesselations [Ha1].
In addition to Escher, other artists have created hyperbolic patterns
during the past few decades.
Some, including Escher, used
“classical”
straightedge and compass constructions (described in [Go1]).
One such artist is Ruth Ross, who created patterns using
different kinds of sea shells.
Other artists used computer methods, such as those used to generate the
patterns of this essay [Du1].
Helaman Ferguson realized the {7,3} tessellation in the stone base
for his sculpture Eight fold Way.
He has also created a leather quilt and a printed pattern
Big Red 5 using the {5,4} tessellation.
For more on Ferguson's work, see his web site
http://www.helasculpt.com/gallery/index.html
[Fe1].
Irene Rousseau has also used the {5,4} tessellation to create a precise
mosaic pattern.
Jan Abas used the {6,4} tessellation in his Islamic star pattern,
Hyperbolic Mural [Ab1].
Craig Kaplan has written a general program that generates Islamic star patterns
in each of the classical geometries [Ka1].
Tony Bomford used a mixture of classical and computer methods in
creating several hooked rugs based on the {5,4} and {6,4} tessellations.
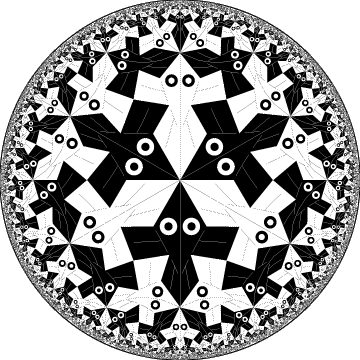
Escher quite successfully overcame all of his criticisms of
Circle Limit I
in his print Circle Limit III, which has 4-color symmetry.
It is based on the {8,3} tessellation, as is shown in Figure 5 below.
Note that the noses and left fin tips of the fish are at alternate
vertices of the octagons.
In describing the print to Coxeter, Escher wrote:
“As all these
strings of fish shoot up like rockets from infinitely far away,
perpendicularly from the boundary, and fall back again whence they
came, not one single component ever reaches the edge.”
([Co2] page 20).
The white backbones of each stream of fish make prominent arcs
on the print and it is tempting to guess that these arcs are
hyperbolic lines (i.e. circular arcs perpendicular to the bounding
circle). Escher's remark might be interpreted to mean this. But this
is not the case. As Coxeter discovered, careful measurements of
Circle Limit III show that all the white arcs make angles of
approximately 80 degrees with the bounding circle. This is correct,
since the backbone arcs are not hyperbolic lines, but equidistant
curves, each point of which is an equal hyperbolic distance from a
hyperbolic line [Co2], [Co3].
In the
Poincaré
model, equidistant curves are represented by circular arcs that
intersect the bounding circle in acute (or obtuse) angles.
Points on such arcs are an equal hyperbolic distance from the
hyperbolic line with the same endpoints on the bounding circle.
For any acute angle and hyperbolic line, there are two equidistant curves
(“branches”),
one on each side of the line, making that
angle with the bounding circle [Gr1].
Equidistant curves are the hyperbolic analog of small circles in
spherical geometry. For example, every point on a small circle of
latitude is an equal distance from the equatorial great circle;
and there is another small circle in the opposite hemisphere the same
distance from the equator.
Each of the backbone arcs in Circle Limit III
makes the same angle A with the bounding circle.
Coxeter [Co2] used hyperbolic trigonometry to show that
A is given by the following expression:
One can imagine a three-parameter family (k,l,m) of
Circle Limit III fish patterns
in which k right fins, l left fins, and m noses
meet, where m must be odd so that the fish swim head to tail.
The pattern would be hyperbolic, Euclidean, or spherical depending on
whether
1/k + 1/l + 1/m
is less than, equal to, or greater than 1.
Circle Limit III would be denoted (4,3,3) in this system.
Escher created another pattern in this family, his Euclidean
notebook drawing number 123, denoted (3,3,3), in which each fish
swims in one of three directions [Sc1]. All the fish swimming in one
direction are the same color.
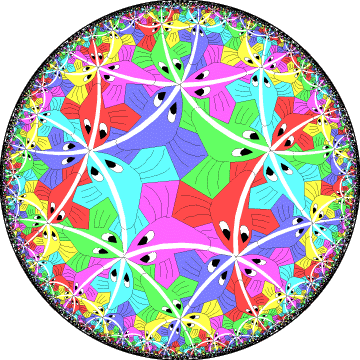
The pattern on the 2003 Math Awareness Month poster is (5,3,3) in this system,
and is shown below in Figure 6.
It is necessary to use six colors to color the lines of fish symmetrically
and adhere to the map-coloring principle: no adjacent fish should be the
same color.
Following Coxeter's calculation [Co2], it is easy to show that the angle
A between the backbones and the bounding circle is given by:
The 2003 Math Awareness Month poster design is just one example of the
connection between mathematics and art.
Of course there are numerous other connections, including those
inspired by Escher in the recent book
M.C.Escher's Legacy [Sc2].
My article [Du3] and electronic file on the CD Rom that accompanies
that book contain many examples of computer-generated
hyperbolic tessellations inspired by Escher's art.
For more on Escher's work, see the Official M. C. Escher Web site
http://www.mcescher.com/ [Es1].
[Ab1] Abas, S. Jan,
Web site:
http://www.bangor.ac.uk/~mas009/part.htm
[Bo1] Bool, F.H., Kist, J.R., Locher, J.L., and Wierda, F., editors,
M. C. Escher, His life and Complete
Graphic Work, Harry N. Abrahms, Inc., New York, 1982.
ISBN 0-8109-0858-1
[Co1] Coxeter, H. S. M.,
“Crystal symmetry and its generalizations,”
Royal Society of Canada(3), 51 (1957), 1-13.
[Co2] Coxeter, H. S. M.,
“The non-Euclidean symmetry of Escher's
Picture `Circle Limit III',”
Leonardo, 12 (1979), 19-25, 32.
[Co3] Coxeter, H. S. M.,
“The Trigonometry of Escher's Woodcut 'Circle
Limit III',” The Mathematical Intelligencer, 18 no. 4 (1996)
42-46. Updated and corrected version appears in [Sc2] below.
[Co4] Coxeter, H. S. M.,
“Angels and devils,” in
The Mathematical Gardner, David A. Klarner, editor,
Wadsworth International, 1981 (out of print). ISBN 0-534-98015-5
[De1] Deraux, Martin, Interactive tessellation web site:
http://www.math.utah.edu/~deraux/tessel/
[Du1] Dunham, D.,
“Hyperbolic symmetry,”
Computers and Mathematics with Applications,
Part B 12 (1986), no. 1-2, 139-153.
[Du2] Dunham, D.,
“Transformation of Hyperbolic Escher Patterns,”
Visual Mathematics (an electronic journal),
1, No. 1, March, 1999.
[Du3] Dunham, D.,
“Families of Escher Patterns,” in [Sc2] below, pp. 286-296.
[Es1] Official M. C. Escher Web site, published by the
M.C. Escher Foundation and Cordon Art B.V.
http://www.mcescher.com/
[Fe1] Ferguson, Helaman,
Web site:
http://www.helasculpt.com/gallery/index.html
[Go1] Goodman-Strauss, Chaim,
“Compass and straightedge in the Poincaré disk,”
Amer. Math. Monthly, 108 (2001), no. 1, 38-49.
[Gr1] Greenberg, Marvin, Euclidean and Non-Euclidean Geometries,
3rd Edition, W. H. Freeman and Co., 1993. ISBN 0-7167-2446-4
[Ha1] Hatch, Don, Hyperbolic tessellations web site:
Hyperbolic Planar Tesselations [Ha1].
[He1] Henderson, David W., and Daina Taimina,
Experiencing Geometry: In Euclidean, Spherical and Hyperbolic Spaces,
2nd Ed., Prentice Hall, 2000. ISBN 0130309532
Web link:
http://www.mathsci.appstate.edu/~sjg/class/3610/hen.html
[Jo1] Joyce, David, Hyperbolic tessellations web site:
http://aleph0.clarku.edu/~djoyce/poincare/poincare.html
[Ka1] Kaplan, Craig S.,
“Computer generated Islamic star patterns,”
Bridges 2000, Mathematical Connections in Art, Music and Science.
Winfield, Kansas, USA, 28-30 July 2000. ISBN 0-9665201-2-2
Web link:
Abstract and PDF
[Ma1] Magnus, Wilhelm, Noneuclidean Tesselations and Their Groups,
Academic Press, 1974. ISBN 0-12-465450-9
[Sc1] Schattschneider, Doris, Visions of Symmetry: Notebooks, Periodic
Drawings, and Related Work of M. C. Escher,
W. H. Freeman, New York, 1990. ISBN 0-7167-2126-0
[Sc2] Schattschneider, Doris, and Michele Emmer, editors,
M. C. Escher's Legacy: A Centennial Celebration,
Springer Verlag, 2003. ISBN 3-540-42458-X
Hyperbolic Geometry and Hyperbolic Art
Hyperbolic geometry was independently discovered about 170 years ago by
János Bolyai, C. F. Gauss, and N. I. Lobatchevsky [Gr1], [He1].
The hyperbolic plane is the only complete surface with constant
negative curvature.
About 100 years ago David Hilbert essentially proved that there was no smooth
isometric embedding of the hyperbolic plane into Euclidean 3-space, so we
must rely on mathematical models to view it [He1].
These models must, perforce, distort distances and possibly angles.
One such model, the Poincaré disk model, is useful to artists
since it is conformal (angles have their Euclidean measure) and it
is displayed in a bounded region of the Euclidean plane, so that
it can be viewed in its entirety.
Circle Limit III and the Poster Design
Escher had several criticisms of his Circle Limit I pattern.
First, the fish are
“rectilinear”, without the curved outlines of
real fish. Also, there is no
“traffic flow” along the backbone lines
— the fish change directions after two fish, and the fish change
colors along lines of fish ([Co2] page 20).
Another criticism, which Escher didn't make, is that the black and
white fish are not equivalent (the nose angles are different),
so there is no color symmetry.
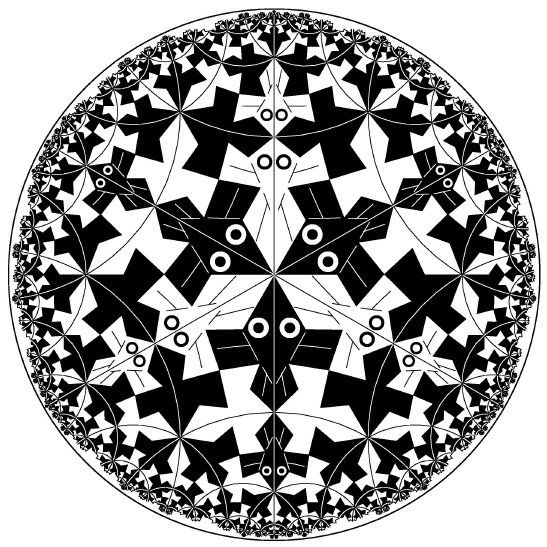
Escher's latter criticisms could be overcome by basing the
fish pattern on the {6,6} tessellation, as shown in Figure 4 below.
In fact Figure 4 can be recolored in three colors to give it
color symmetry, which means that every symmetry (rotation, reflection, etc.)
of the uncolored pattern exactly permutes the colors of the fish in the
colored pattern [Du2].

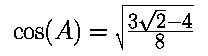
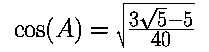
References
Republished as:
Mathematical Recreations: A Collection in Honor of Martin Gardner,
David A. Klarner, editor, Dover Publishers, 1998. ISBN 0-486-40089-1
Page URL: http://www.d.umn.edu
/~ddunham/mam/essay1.html
Page Author: Doug Dunham
Last Modified: Monday, 03-Feb-2003 20:22:37 CST
Comments to: ddunham@d.umn.edu