Classroom Examples for Test 2

Example #1
A 41 kg box is pulled across a frictionless floor by a rope which makes an angle of 34.2^o with the horizontal and applies a force of 22.2 N. What is the acceleration of the box across the floor?

Example #2
A 17.0 kg bucket is lowered by a rope with a constant velocity of 0.500 m/s. What is the force exerted by the rope on the bucket (the tension in the rope)

Example #3
A 10.6 kg bucket is raised with a constant upward acceleration of 1.00 m/s². What is the force exerted by the rope on the bucket?

Example #4
A 10.6 kg bucket is lowered with a constant downward acceleration of 1.00 m/s². What is the force exerted by the rope on the bucket?

Example #5
Rose is sledding down an ice-covered hill inclined at an angle of 15^o with the horizontal. What is the acceleration of Rose and her sled down the hill?

Example #6
A 4.00 kg block is pushed along the ceiling with a constant applied force of 85.0 N that acts at an angle of 55.0^o with the horizontal. The block accelerates to the right at 6.00 m/s². Determine the coefficient of kinetic friction between block and ceiling.



Example #7
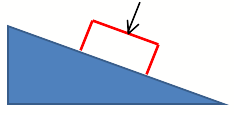
The coefficient of static friction between the 3.00 kg crate and the 35^o incline is 0.300. What minimum force F must be applied to the crate perpendicular to the incline to prevent the crate from sliding down the incline?

Example #8
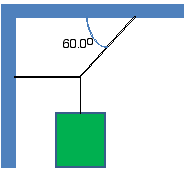
A box hangs from the wires as shown. What is the tension in each wire if the box weights 20.0 N.

Example #9
A 5.00 kg block rests on a level table. The coefficient of friction between the block and the table is 0.55. A 4.00 kg weight is attached to the block by a string of negligible mass passed over a light frictionless pulley. What is the acceleration of the block when the 4.00 kg weight is released?

Example #10
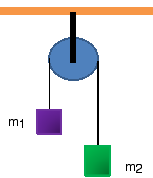
A classroom demonstration is done with an Atwood machine. The masses are m₁ = 1.00 kg and m₂ = 1.10 kg. If the larger mass descends a distance of 3.00 m from rest in 3.6 s, what is the acceleration of gravity at that place?

Example #11
A child sits on a rotating merry-go-round, 2.1 meters from its center. If the speed of the child is 1.9 m/s, what is the minimum coefficient of static friction between the child and the merry-go-round that will prevent the child from slipping?

Example #12
A 0.255 kg ball tethered to a tall pole on a 1.37 m rope is thrown so that it travels in a horizontal circle with the rope making an angle of 40.0^o with the vertical pole. a) What is the speed of the ball? b) What is the tension in the rope?

Example #13
a) Find the orbital speed of a satellite in a circular orbit 1700 km above the surface of the Earth. b) What is the period of this orbit? The mass of the earth is 5.97 x 10²⁴ kg and the radius of the earth is 6370 km.

Example #14
A luggage handler pulls a 20.0 kg suitcase up a ramp inclined at 25.0^o above the horizontal by a force F of magnitude 141 N that acts parallel to the ramp. The coefficient of kinetic friction between the ramp and the incline is .300. If the suitcase travels 3.80 m along the ramp, calculate a) the work done on the suitcase by the force Fb) the work done on the suitcase by the normal force.c) the work done on the suitcase by the gravitational force.d) the work done on the suitcase by the friction force.e) the total work done on the suitcase.

Example #15
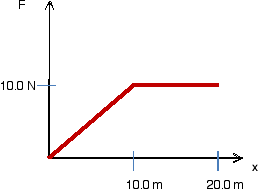
A net external force is applied to a 6.00 kg object that is initially at rest. The net force component along the displacement of the object varies with the magnitude of the displacement as shown in the drawing.a) How much work is done by the net force?b) What is the speed of the object at d = 20.0 m?



Example #16
The cheetah is one of the fastest accelerating animals, for it can go from rest to 27 m/s (about 60 mi/h) in 4.0 s. If its mass is 110 kg, determine the average power developed by the cheetah during the acceleration phase of its motion.

Example #17
A cyclist approaches the bottom of a gradual hill at a speed of 11.0 m/s. The hill is 5.0 m high, and the cyclist estimates that she is going fast enough to coast up and over it without pedaling. Ignoring air resistance and friction, find the speed at which the cyclist crests the hill.

Example #18
A 2.0 x 10³ kg car moving at an initial speed of 25 m/s along a horizontal road skids to a stop in 60.0 m. a) Find the energy dissipated by friction.b) Find the coefficient of kinetic friction between the tires and the road.

Example #19
(#96 page 250) A block of mass m slides from rest on a frictionless loop-the-loop track. What is the minimum release height, h, required fro the block to maintain contact with the track at all times?

Example #20
(#93 page 250) An ice cube is placed on top of an overturned spherical bowl of radius r. If the ice cube slides downward from rest at the top of the bowl, at what angle q does it separate from the bowl?

Example #21
A box is given an initial speed of 4.0 m/s up a 20.0^o incline. The coefficient of kinetic friction between the box and the incline is 0.20. How far up the incline does the sled move? Use energy methods to solve.

Example #22
Two blocks, stacked on top the other, can move without friction on the horizontal surface shown. The surface between the two blocks is rough, however, with coefficient of static friction equal to 0.47.a) If a horizontal force F is applied to the 5.0 kg bottom block, what is the maximum value F can have before the 2.0 kg top block begins to slip?b) If the mass of the top block is increased, does the maximum value of F increase, decrease, or stay the same? Explain.